How to calculate the area of \u200b\u200bthe rhombus?

In this article, you will learn how to find a rhombus area with various methods. Thanks to these formulas, it will be easily solved by geometry problems, because here the article will describe how to calculate the area of \u200b\u200bthe rhombus, knowing the size of the diagonal of the larger and smaller, side, angles and diameter of the inscribed circle in rhombus.

You can find out the area of \u200b\u200bthe rhombus by different formulas. It is enough to know the properties of this figure and properties of other figures, because the rhombus can be divided into triangles, it can be equated with a parallelogram, etc. Below you will see such formulas. You also need to know how the rhombus and a parallelogram differ. According to the mathematical definition. The rhombus is a figure of such a parallelogram with equal sides, but unlike a square - the rhombus has no straight angles. But the sum of the two angles at the base of the rhombus will be 180 degrees. All this knowledge will come in handy for calculating the area of \u200b\u200bthe rhombus, then in more detail.

How to calculate the area of \u200b\u200bthe rhombus - the properties of the figure

Before calculating the area of \u200b\u200bthe rhombus, it is better to familiarize yourself with the properties of this figure. Indeed, thanks to knowledge of these characteristics, it will be easier to prove the probability of a particular formula. Earlier it was already mentioned what rhombus is. It is a figure with equal to all sides equal to opposite sharp and dumb corners, but not straight.

The rhombus has the following properties:

• all sides are equal to each other
• the corners lying opposite each other are also equal
• the diagonals of this figure are bisectors, at the intersection point are divided into equal segments
• the diagonals also intersect in the center of the rhombus and at right angles
• the opposite sides of the figures cannot intersect, even if you extend the rays, they are parallel, like a parallelogram.

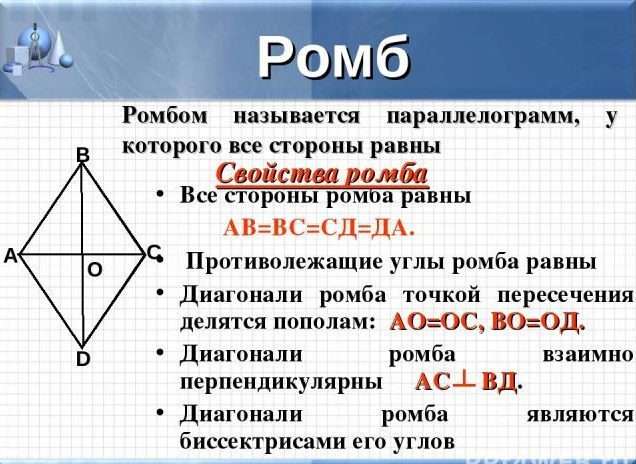
IMPORTANT: Please note that the rhombus can be divided into four rectangular triangles, which will be equal in area, or by two equilateral identical triangles, see the image above.

How to calculate the area of \u200b\u200bthe rhombus?

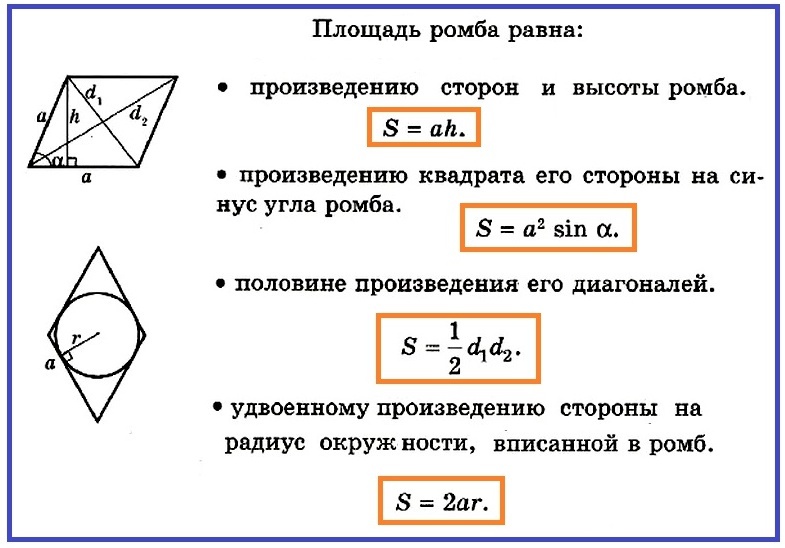
So, let's find out how the rhombus area is calculated. Let's take advantage of the formula for the area of \u200b\u200bthe rectangle, where:

• S \u003d A • Bwhere a, b are the sides of the rectangle.

To make it clear how to get a rhombus formula from this formula, the formula of the area, see explanation:

1. Draw a rhombus, make a height to the base of the BH rhombus.
2. From point D to the AD line, also draw a CH1 height.
3. It turns out that the triangle ABH and the triangle CH1D are equal to each other on two common sides, ∠ COVER between them.
4. So AH \u003d DH1. The area of \u200b\u200bthe resulting square will be equal to the area of \u200b\u200bthe rhombus
5. So BH • HH1 is the area of \u200b\u200bthe rhombus, in other words, the work of the BH romb altitude to the side of AD and will be s area of \u200b\u200bthe rhombus, since HH1 \u003d BC, and BH is the height.

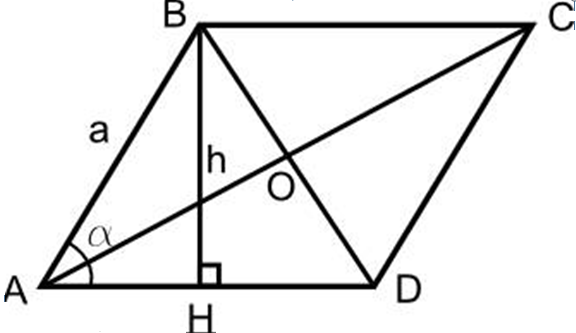
It follows from the proof that:

• S rhombus \u003d a • H and measured in square units.

How to find the area of \u200b\u200bRomb, knowing the corner and side of the geometric figure?

Now we know what the formula of the rhombus area looks like, we can find the rhomba area on the same formula, knowing what the side of the rhombus is equal to the side of the rhombus, for example, sharp at the base, as in the photo below.

• S \u003d A • H

But in our case, we are unknown to the height of the rhombus, it should be found. To do this, you will have to consider a rectangular triangle, which turned out when height was drawn to the base of the rhombus.

In this triangle is known hypotenuse and ∠α. To calculate the area of \u200b\u200bthe entire figure, you will need to find a height. A h \u003d a • sin. So s the area of \u200b\u200ban equilateral parallelogram (rhombus) is:

• S \u003d A • A • Sin They ax \u003d A² • Sin.

How to calculate the area of \u200b\u200bthe rhombus, knowing its diagonals?

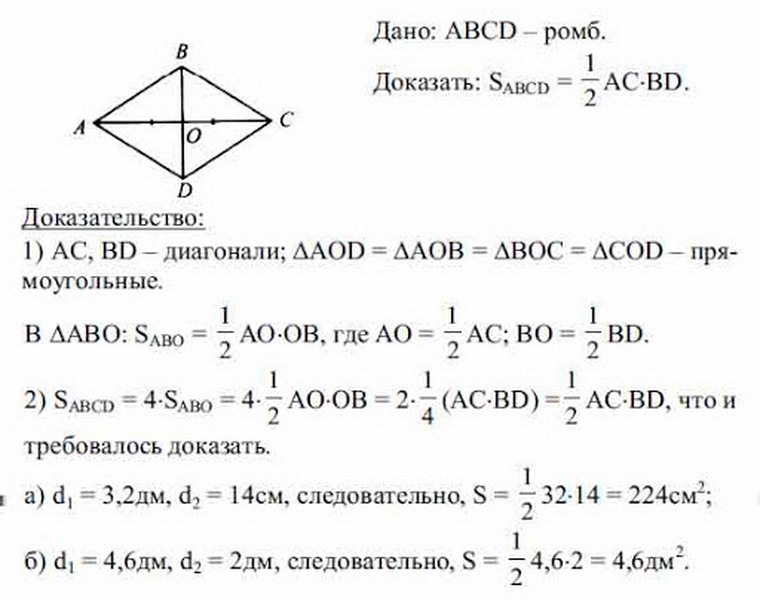
To find out the formula of the area of \u200b\u200bthe rhombus, when only (a, b) diagonals are known, the following example should be considered. BCDA is given - rhombus and know what the diagonals are equal. Now it is necessary to find s area of \u200b\u200ban equilateral parallelogram in terms of diagonals.

Previously, the properties of the rhombus were already considered. The diagonals of the rhombus are equal, at the intersection point they are divided into equal segments. From this it follows that all the triangles that are inscribed in the figure as a result of the intersection of both diagonals are also equal to each other and they are rectangular (on three sides). To find the area of \u200b\u200bthe rhombus, it is enough to find the area of \u200b\u200bone triangle and multiply the data obtained by 4.

In total, it turns out that:

• S rhombus \u003d 4 (1/2 AO • OB + 1/2 BO • OC + 1/2 OC • OD + 1/2 OD • AO) \u003d 4 • 1/8 AC • BD \u003d 1/2 BD • AC, Total Square S Romm will be \u003d work a • b (diagonals) divided by two: S \u003d 1/2 A • B

How to calculate the area of \u200b\u200bthe rhombus, knowing its side and the radius of the circle inscribed in it?

The rhomba area can be calculated by knowing R - radius and a - the length of the side of the figure. It is already known that S - the area of \u200b\u200bthe figure will be equal to the work B - the side on h - height. Through the center of the circle, it will also be the intersection center A, B is the diagonals of the rhombus. Carry out the height and at the same time the diameter of the rhombus. The image shows that the height of the figure is two radii of the circle. Now it will be easy to find the area of \u200b\u200bthe rhombus itself:

• S \u003d A • H \u003d A • 2R

Below, see the example of the task on this topic.

Still see such articles on this topic here:

1. The area of \u200b\u200bthe rectangle, how to find?
2. How to find a circle area?
3. Square area - formulas.

Video: an example of how to calculate the area of \u200b\u200bthe rhombus